Achieve Good Packed Tower Efficiency
Many plants rely on direct-contact mass transfer operations such as distillation, absorption and stripping. Indeed, overall process performance frequently rests heavily upon the efficiency of the mass transfer, which, in turn, depends upon tower design.
Design of a direct-contact mass transfer column must consider two major components. One is the number of theoretical stages (TS) or transfer units required for the separation. (In this article, TS refers to both.) The second is the mechanical selection and design of the tower internals (packing or trays). These two are intimately tied together through the question: What depth of packing or how many trays will provide the number of TS required? The answer depends upon the efficiency of the contacting device and that’s influenced by its mechanical design.
So, we’ll explore the effect of liquid distributor design on the performance of towers using random or structured packing. The number of TS required is calculated with a given liquid-to-vapor (L/V) ratio. Any deviation from this ratio caused by poor (uneven) liquid distribution negatively impacts the separation. This lack of separation often is misinterpreted as poor packing efficiency, that is, a higher-than-expected height equivalent to a theoretical plate (HETP). However, the packing’s mass transfer capability didn’t change — poor liquid distributor design caused a change in L/V ratio and thus the loss in separation efficiency.
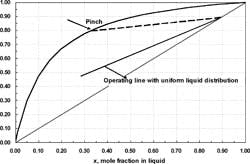
Figure 1 -- Impact of L/V ratio: Change in ratio can lead to an equilibrium pinch in which no further separation can occur.
The deviation from the desired L/V ratio in some areas of the tower may result in equilibrium pinching — a condition in which the packing won’t appear to be working because further separation can’t occur no matter what depth of packing is available (Figure 1). Over its efficient operating range, the packing should provide a relatively constant level of vapor/liquid contact and therefore nearly identical interfacial area for mass transfer. So, when you suffer loss of packing efficiency indicated by the lack of separation, focus on a change in L/V ratio due to liquid, or less commonly vapor, maldistribution.
Liquid Distribution
All liquid distributors use an orifice to meter the liquid onto the top of a packed bed. Liquid from the orifice may enter a pipe to avoid being entrained by rising vapor or may impinge upon a spreader plate to improve distribution. The driving force across the orifice is provided either by a static head (from a pool or a standpipe pool of liquid) in a gravity liquid distributor or by pressurized liquid (normally from a pump). Figures 2–4 show various types of liquid distributors.
Figure 2 -- Trough arm distributor: Water pours out of bottom orifices of Sulzer VKG on test stand at FRI.
Uniform liquid distribution is key to obtaining expected performance from a packed bed. The distributor needs to uniformly allocate the liquid for all anticipated flow rates, with an adequate number of pour points for the size of the packing and sufficient open area for vapor passage. Both liquid orifice point-to-point flow uniformity and uniformity of the orifice drip-point pattern across the superficial tower area are crucial to obtaining good packing performance.
Liquid maldistribution affects packing performance in two ways. The local variations, orifice to orifice, in terms of the L/V ratios may cause the compositional pinch shown in Figure 1. And liquid maldistribution over a large section of the tower cross-sectional area may prompt the liquid to flow unevenly through the packing, concentrating at the wall.
Figure 3 -- Another trough arm distributor: This Raschig DTS trough arm distributor features side orifices with discharge tubes.
When a liquid distributor is operated at rates below its designed turndown ratio, it won’t provide uniform flow point-to-point and across the tower cross-sectional area because of low liquid head above the orifices. As the flow uniformity diminishes the separation deteriorates.
Weir flow and notched trough distributors generally give poor liquid distribution. They also are susceptible to creating liquid entrainment — because they discharge liquid into the vapor riser area where vapor velocity typically is three times higher than the superficial tower vapor velocity even when the packed bed is running at reasonable rates — that can lower separation efficiency by liquid back-mixing. However, this type of distributor may be a good choice if plugging and fouling are a serious problem.
Figure 4 -- Pan distributor: Bottom view shows details of FRI TDP pan distributor.
The spray nozzle distributor poses a similar entrainment problem because it breaks the liquid up into small droplets that can be easily carried back up the tower. But, in this case, no restrictive vapor passage exasperates the entrainment potential.
Key criteria
A well designed liquid distributor should offer:
• uniformity of flow;
• appropriate pour-point density;
• proper irrigation along the column wall area;
• sufficient open area for vapor flow; and
• entrainment prevention.
The degree of the uniformity necessary depends upon the service. For example, applications for mass transfer or heat transfer with a close approach to equilibrium require higher flow uniformity than those for general heat transfer purposes. When flow variation is measured by the standard deviation of liquid flow through the pour points, the ratio of the standard deviation to mean liquid flow rates, Cv, should be limited to ± 5% [1].
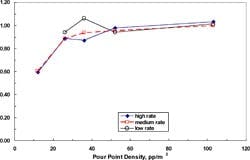
Adequate pour-point density is important to achieve the optimum efficiency for any packing. A pour point density as low as 3 points/ft2 may yield satisfactory results for some packings [2] (Figures 6 and 7). Packings with high surface area may require a greater pour-point density (>10 points/ft2). We suggest using 6–9 points/ft2 for metal Pall rings.
Figure 6 -- Structured packing: Pour point density causes significant differences in packing efficiency, according to FRI data.
Liquid irrigation along the column wall also is important. Too large a distance between the column wall and the outer distributor pour points (e.g., when a distributor is set on top of a tray ring) cuts the efficiency of the packing. Too many pour points near the column wall may lead to over-irrigation there, resulting in poor separation efficiency because of pinching in some areas of the bed that are under-irrigated or short-circuiting liquid down the tower wall past the packing and the rising vapor [2 ,3].
The distance between the bottom of the liquid distributor and the top of the packing, as well as the distributor open area, must be chosen to avoid liquid entrainment due to high vapor velocity. Typical practice is to put the distributor or redistributor six inches above the bed. The closer the tower is operated near the maximum capacity of the packing, the more care a designer must take to ensure that the liquid streams leaving the distributor don’t break up into droplets and get carried up the vapor risers.
Figure 7 -- Pall rings: FRI data indicate the impact of pour point density on the efficiency of 1-in. rings.
A typical distributor has about 33% open area, so the velocity of the vapor entering the riser area is about three times that of the vapor leaving the top of the packed bed. Some specially designed distributors offer open areas as high as 45%. Liquid that goes up risers and then falls on the distributor can prompt premature distributor flooding by increasing the distributor’s liquid load. Liquid entrainment between packed beds will cause liquid back-mixing and decrease separation in the bed above. If there’s no bed above, the entrainment will be carried out of the tower, lowering the purity of the overhead product.
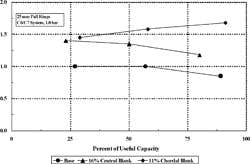
For optimum packing performance it’s crucial to avoid large-scale or zonal maldistribution [2]. A large-scale maldistribution pattern will more severely lower efficiency than a small-scale random liquid maldistribution. Figure 8 shows the significant impact of blanking off a central area and a chord of the distributor. The height of packing required to even out concentration differences can vary substantially depending upon the severity of maldistribution and the size of the packing. Maldistribution affects large packings (> 2.5 in.) less than small ones. Slight random variations in flow through the recommended number of pour points uniformly distributed over the tower cross-section should minimally impact performance. However, flow variations that are localized or zonal in nature could undermine performance [3].
Figure 8 -- Zonal maldistribution: Blanking off portions of a distributor to create maldistribution lowers packing efficiency as shown by FRI data.
Other Considerations
The liquid distributor often is the limiting factor in turndown unless it’s specifically designed for a high turndown, for example using multi-level liquid orifices. The equation for flow through an orifice (Eq. 2 below) governs the design of the distributor. This means that flow is proportional to the square root of liquid head; so, for example, a turndown of 4:1 would translate into an increase of 1,600% in liquid elevation above a hole. It’s clear that turndown requirements demand careful consideration to avoid tall and expensive liquid distribution devices.
Because separation efficiency tends to deteriorate with increase in packed bed depth, liquid should be redistributed approximately every 30 ft to 35 ft or every 20 TS per bed, whichever is lower. (Some studies indicate that a greater number of TS are possible without the efficiency decrease [4].) When it’s necessary to satisfy structural strength requirements due to bed weight or to introduce feed or withdraw side streams, a liquid redistributor should be used. Redistributors must be placed between beds — and designed and installed as carefully as distributors at the top of the bed. The main difference between a reflux or primary distributor and a redistributor is that the redistributor is between packed beds and therefore has liquid falling on it from the bed above; so it requires vapor riser covers to prevent the falling liquid from entering the risers and being entrained up the tower.
The distribution of vapor entering the bottom of a packed bed normally isn’t as great a concern as liquid distribution. However, vapor must be reasonably evenly distributed before it enters the bottom of the bed. Adequate vapor distribution can be a concern especially when a tower is converted from trays to packing. The pressure drop per theoretical packed stage is one-tenth to one-fifth that of a typical tray, so it’s important to make sure that the kinetic energy of the incoming vapor is dissipated sufficiently by the packed bed resistance or a vapor distributor/diffuser. Refs. 1 and 5 provide design considerations for this. The resistance necessary for vapor distribution should be provided by about one TS of packing. If it isn’t, consider a vapor distributor — otherwise pinching effects as discussed above may reduce separation efficiency.
Flow variation
This can occur on both micro and macro scales. Micro flow variation is the flow differences among orifices and may be expressed by Cv, as suggested in Refs. 2 and 6. Macro flow variation is between significant distributor areas, which is defined in Ref. 1 as one-twelfth of the tower area. Ref. 7 provides a detailed discussion of liquid distributor evaluation.
A number of factors can cause flow variation:
• shape of the orifice;
• shape of the edge of the orifice;
• orifice layout pattern;
• orifice density;
• depth of the liquid pool above the orifice;
• horizontal liquid velocity above the orifice;
• velocity through the orifice; and
• feed pipe design.
All must be considered when designing or reviewing the design of a liquid distributor.
Shape of the orifice. It’s normally assumed that the orifice is round. The flow through the orifice is a function, among other things, of the ratio of the orifice perimeter to the open area, which is incorporated in the orifice coefficient. Standard industrial practice is to punch, not drill, the liquid distributor orifices; drilled holes usually are lobed-shaped not round. Non-circular orifices also are employed — in such cases, the hydraulic diameter for the non-circular orifice needs to be used for the distributor design and flow capacity calculation, etc.
Shape of the edge of the orifice. The orifice is assumed to be sharp-edged. Depending upon the edge condition, the direction of flow relative to the punched direction, and the ratio of plate thickness to orifice diameter, the orifice coefficient will vary from about 0.52 to 0.98 [8]. Liquid flow always should be in the direction of the punching [8]. The punch creates a sharp edge as it enters and a torn rounded edge as it passes through the material. If good quality control isn’t maintained during punching operations, the orifice shape and, consequently, the orifice coefficient will change from the beginning to the end of manufacturing. This will result in the orifice coefficient not matching the design, which may cause a problem in tightly designed distributors at the low or high liquid rate extremes of the operating range. For long production runs, this could lead to macro maldistribution, which we’ll address later. Micro flow variations among orifices have little effect on random packing efficiency if the orifices with the variations are randomly distributed across the tower area. Similar variations do have an effect on structured packing efficiency [2, 3].
Orifice layout pattern. This should ensure that liquid entering the top of the packed bed is evenly distributed across the tower cross-sectional area. Liquid streams leaving the distributor shouldn’t impinge upon or be interfered with by the bed limiter. Don’t rely on the packing to markedly improve initial liquid maldistribution. The packing can enhance liquid distribution on a micro scale but not on a macro scale. The spreading coefficient of the packing may suffice to spread the liquid 6 in. to 12 in. from its entry point on the top of the bed but it can’t even out the liquid flow for several feet or more across the tower. If the L/V ratio is wrong, while the packing is evening out liquid flow the desired separation won’t be achieved unless a large safety factor is applied to allow for this decrease in apparent packing efficiency.
Bunching the orifice together to make room for a gas riser or a tray ring can cause micro maldistribution. The methods for laying out the liquid orifice pattern differ slightly for random packing [1] and structured packing [9] because random packing spreads liquid in the shape of an inverted cone, 360° spreading, while structured packing spreads liquid more in one direction than the other and depends upon the rotation between adjacent layers to get 360° liquid spreading.
Orifice density. The number of orifices per unit area affects packing efficiency. With too few, liquid is essentially maldistributed on a micro scale because orifices are so far apart that a large portion of packing remains un-wetted, resulting in poor interfacial contact area, improper L/V ratios and vapor bypassing. However, using a few large orifices does somewhat decrease the cost of a distributor and the problem of fouling. On the other hand, using many small orifices will boost separation efficiency but also the cost of the distributor and the chance of plugging. Generally it’s best to keep the density between 4 and 10 points/ft2 [2]. The use of spreader plates on the orifice discharge allows a lower orifice count while maintaining good distribution [9].
Depth of the liquid pool above the orifice. In a gravity head distributor this is the sum of the two components: hOA = hO + hPD (1) where hOA is the total liquid head in the liquid distributor, in.; hO is the orifice head, in.; and hPD is the distributor head loss on the vapor side, in. It is good practice to add one to two inches to hOA when determining the physical height of the vapor risers to prevent liquid from entering the vapor risers at maximum flow rate.
The flow through the orifice is defined [8] as: QO = 19.636 Kd2hO
The head required for flow through n orifices in a distributor can be calculated by rearranging Eq. 2 and changing hO to inches: hO = 0.0311 QT2/K2d4n2 (3)
Rearranging Eq. 3 gives the number of orifices required for a specified head: n = 0.1764 QT/Kd2hO0.5 (4)
where QT is total liquid flow to distributor, gpm.
It can be seen from Eq. 2 that the flow, if all else is held constant, is a square root function of liquid head above the orifice. Therefore, at low heads (< 1 in.) the flow is very sensitive to small changes in liquid depth. A ±¼-in. variation in head changes flow +11.8% to -13.4%. Thus, an out-of-level distributor with a 1-in. deep pool having a ¼-in. wave action will have 25.2% flow variation. If the pool depth is increased to 2 in. the same ¼-in. head variation will be reduced to +6.1% to -6.5% for a maximum variation of 12.6%. Thus, a minimum liquid pool depth of 2 in. is in line with the recommendation in Ref. 1 of an orifice-to-orifice variation of ±5%.
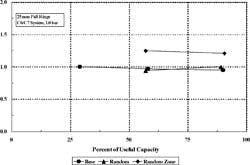
Figure 9 -- Point versus zonal maldistribution:
The nature of liquid maldistribution, whether random or random zonal, changes the impact on packing efficiency.
For random packing, Fractionation Research, Inc. (FRI) found that a flow variation of ±12.5% randomly spread across the tower cross-sectional area didn’t degrade the efficiency of 1-in. metal Pall rings. However, if the flow variation isn’t randomly spread across the tower but isolated in zones where all low-flowing orifices are in one area and high-flowing orifices in another area, the apparent packing efficiency declined by 20% [2] (Figures 8 and 9). For structured packing the results were similar but the apparent packing efficiency declined for both the distributed random flow variation and the zonal maldistribution distribution [2].
Effects of liquid velocity through the orifice. The orifice discharge coefficient also is affected by the liquid velocity through the orifice. It depends on the Reynolds number based on the orifice diameter. Most published studies on orifice flow were conducted for orifice meters [10]. When a fluid flows through a thin-plate orifice the jet contraction amounts to about 60% of the orifice area, so orifice discharge coefficients are on the order of 0.62 at Reynolds numbers above 10,000, with a maximum of about 0.7 occurring at about a Reynolds number of 200 compared with nearly unity for venture tubes and flow nozzles. The actual coefficient also is a function of the ratio of the orifice to the pipe sizes and Reynolds number. Very little published data exist on actual orifice coefficients for distributors. In the case of a packed tower distributor the orifice is discharging into a nonrestrictive open space that can be considered an infinite pipe diameter, so Eq. 2 can be used without correction for the ratio of the orifice diameter to the pipe diameter because here the ratio is less than 0.3 [8].
As part of a comprehensive research program on liquid flow through distributors FRI found that even when flow through the orifice is in the fully turbulent regime the orifice coefficient isn’t constant and the liquid head above the orifice is a critical parameter in determining the orifice discharge coefficient. At sufficiently low liquid head the orifice flow becomes unstable for a number of reasons. For instance, wave action in the distributor affects the head on the orifice more at low liquid pool depths than at higher liquid head levels, as we’ve already explained. Also, when the liquid pool is too low the orifice isn’t operating as a metering orifice. In addition, FRI has determined that the discharge coefficient of a vertical orifice differs slightly from that of a horizontal orifice. Ref. 7 indicates that the orifice coefficient can be as low as 0.62 and recommends use of a coefficient of 0.707 for punched holes. A good rule of thumb is to design the liquid head at the minimum flow rate to be 2 in. or greater.
Vapor-side pressure drop across liquid distributor. This will increase the liquid head needed to deliver the design flow capacity. Because vacuum columns are sensitive to pressure drop, distributors have to be designed for minimum vapor-side pressure drop. Pressure drop through a distributor is a function of open area, so narrow–trough, high open area distributors are preferred and spray distributors maybe used (where efficiency isn’t important and liquid entrainment isn’t a concern). Thus, gravity distributor design must consider the pressure drop effect on the head requirements.
The vapor-side pressure drop, Δp, is composed of friction losses inside the vapor risers, Δpf, contraction losses in vapor entrance, Δpc, and expansion losses in vapor exit, Δpe: Δp = Δpf + Δpc + Δpe (5)
These component losses can be calculated via the following equations:
Δpf = f (L/Dh)ρvVv2 (6)
Δpc = KcρvVv2 (7)
Δpe = Ke ρvVv2 (8)
where L is the height of the distributor; Dh is the hydraulic diameter of the risers; Vv is the vapor velocity; Kcis the contraction resistance coefficient; Ke is the expansion resistance coefficient; and ρv is the vapor density. From the contraction and expansion ratios, the Kc and Ke are 0.65 and 0.35, respectively. The hydraulic diameter, Dh, determined by Eq. 8, enables the pressured drop of fluid flowing in irregularly shaped conduits and channels to be calculated as if the fluid were flowing in a round pipe: Dh = 4A/WP (9)
where A is the cross-sectional area and WP is the wetted perimeter of the riser.
The friction factor, f, is computed using the Colebrook equation for turbulent pipe flow:
(10) where ε is the relative roughness of the pipe, which is about 0.0018 in. for stainless steel. If the risers are noncircular pipes, the hydraulic diameter, Dh, needs to be used. The vapor Reynolds number, Re, is based on Dh.
The vapor velocity, Vv, in the riser was approximated using weighted vapor rates and riser opening areas. The weighted vapor rate was calculated by multiplying total vapor rate by the ratio of the riser opening area to total opening area of the column cross-section at the distributor level.
The pressure drops calculated from Eq. 5 were converted to head losses via: hPD = Δp/ρLg (11)
Effects of horizontal liquid velocity on orifice flow. The “still liquid pool” above the orifice should be sufficiently deep to prevent orifice flow instability over the expected distributor operating range, as discussed above. The pool should have large enough volume to keep the horizontal velocity low so as not to significantly vary the liquid head above orifices due to the Bernoulli effect. High horizontal liquid velocity in a distributor trough or arm reduces flow through the orifices by lowering the pressure above the orifice in high velocity areas and increasing the head above orifices in areas where the liquid slows down or stops flowing. The head change caused by the horizontal velocity change of the liquid in a trough can be calculated [1] by:
hv = 0.187 Vh2 (12) where hv is the velocity head, in.; and Vh is the horizontal liquid velocity, ft/s.
It’s best to keep the horizontal velocity under 1.25 ft/s at the minimum head condition. The effect of the horizontal velocity also depends on the total liquid head above the orifice, with less impact on distributors with high liquid head.
Feed pipes. The importance of feed pipe design for distributors or redistributors often is underestimated. It’s critical that liquid leaving the feed pipe doesn’t upset the hydraulics in the distributor. It shouldn’t enter the vapor risers or be entrained by the vapor exiting the vapor riser. This can be accomplished if the liquid is discharged below the top of the vapor risers.
The liquid leaving the feed pipe shouldn’t impinge on the orifices of the distributor or parting box. Otherwise it’ll generate high horizontal velocities and waves across the orifices that will cause flow variations out of orifices. Kister [7] recommends keeping the velocity under 10 ft/s, preferably less than 5 ft/s. As tower diameter increases the design of the feed pipe becomes more complicated, especially if it goes directly into the distributor and not a parting box. Ref. 5 gives criteria for designing perforated pipe distributors and suggests a pressure drop across the orifice of ten times the velocity head of the liquid in the feed pipe for a ±5% flow variation among orifices. This is better than what’s required in most applications but is a good design starting point.
Obtain optimum performance
The design of liquid distributors and redistributors plays an important role in the performance of packed towers. To realize the packing’s full mass transfer-efficiency it’s imperative that liquid entering a packed bed is evenly distributed, so it wets the entire packing surface as soon as possible, and is flowing uniformly across the tower area at the same L/V ratio as used in the TS calculation. To achieve this, the designer must pay close attention to the hydraulics of liquid distributors and redistributors. Distribution of vapor entering the bottom of a packed bed whether it’s coming from a vapor inlet or the bed below, although not the focus of this article, deserves equal consideration.
Frank Rukovena is vice president, marketing and sales, for Fractionation Research, Inc., Bartlesville, Okla. Dr. Tony Cai is a senior research engineer for Fractionation Research, Inc., in Stillwater, Okla. E-mail them at [email protected] and [email protected].