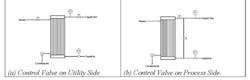
At many plants the performance of steam-heated shell-and-tube exchangers can significantly impact operations. The three previous articles in this series examined regulation of liquid outlet temperature of tubular exchangers via a control valve on the utility side, either on the steam supply or the condensate return. This concluding article looks at the use of one or two control valves on the process side, specifically, to bypass some liquid around the exchanger.
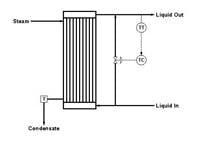
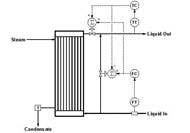
Figure 1. Low cost option: A single control valve
can provide liquid bypass control.
Click image to enlarge.
Figure 2. Operating lines: Equal-percentage valve shows only modest departure from linearity in
both cases. Click image to enlarge
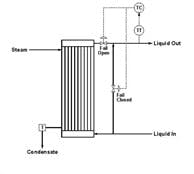
Figure 3. Blocking exchanger flow: This
is the simplest configuration using two
normal control valves.
Click image to enlarge
Figure 4. More complex operating lines: All lines contain significant nonlinear
characteristics, making tuning harder. Click image to enlarge.
Figure 5. Split-range configuration:
When controller output is at mid-range,
both valves are fully open.
Click image to enlarge
Table 1: Split-Range Logic
Controller output (%) |
Exchanger valve position (%) |
Bypass valve position (%) |
0 |
100 |
0 |
50 |
100 |
100 |
100 |
0 |
100 |
Figure 6. Dead zone: Equal-percentage valve likely is preferable despite the flat
region just above mid-range. Click image to enlarge
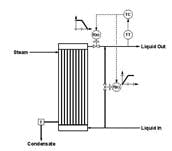
Figure 7. Flow and temperature control: The degree of interaction between temperature and flow
loops depends on the configuration. Click image to enlarge.
Figure 8. Liquid bypass configurations: Two control configurations can accommodate two simple
feedback loops. Click image to enlarge.
Figure 9. Minimizing interactions:
Using summers to determine the
position of each control valve can
reduce the degree of interaction.
Click image to enlarge
About the Author
Cecil L. Smith
Cecil L. Smith
Sign up for our eNewsletters
Get the latest news and updates
Sponsored