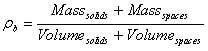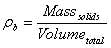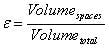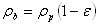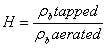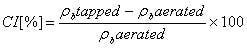Properly accounting for how bulk solids actually will flow in a vessel or overall process can be crucial for successful operations. So, in this article, we will look at two parameters the Compressibility Index and the angle of repose (see sidebar) that can help. While neither provides definitive answers about flowability, they do give rough guidance about how a material is likely to behave.
However, before we discuss these parameters, it is important to understand bulk density. It is probably one of the most common and widely used of the bulk characteristics. It is employed to determine wall loading in hopper design, to size volumetric feeders, such as screws and rotary valves, to estimate flowability, and in many other ways. It is rather unfortunate then that such a useful characteristic is not a constant for a given material. The bulk density of a material is simply the mass of material divided by the volume that it occupies. The density of the particles themselves can be taken as constant; however, the complication arises because the amount of space between the particles depends upon how the material has been handled before the measurement. The volume that a unit mass of product can occupy can differ by 50% between the material being in a compressed and a very loose state. Cement, for example, has a compacted bulk density of 1,400 kg/m3 and an aerated bulk density of 1,000 kg/m3. It is obviously important that the correct bulk density value is selected for any calculation.
Bulk density
The full expression for bulk density is:Measurement of bulk density is, in theory, quite simple; it only requires a knowledge of material mass and volume and is generally based on one of two techniques.
The first is to weigh out a quantity of material using a simple balance and put this into a calibrated cylinder in much the same way as you would a liquid. If the particulate material is poured into the cylinder, the volume taken up would be of the material in a loose or poured state; the associated bulk density is commonly described as poured bulk density. If this same cylinder is then tapped or dropped from a small height onto the bench several times, the volume would likely decrease and the new value is called the tapped bulk density. Similar techniques can be used to determine aerated bulk density from a fluidizing column or compacted bulk density from a material placed under load.
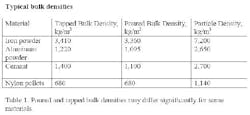
The second technique is to fix the volume of the bulk material by filling a cup-like vessel to overflowing and then leveling it with a straight edge. The vessel then is weighed on a balance and the bulk density calculated. This approach gets around some of the problems of trying to estimate the actual level of powder in a cylinder with a surface that typically is anything but flat and seeing through a glass that has become coated in powder. Table 1 lists typical bulk density values for a few common materials.
Click to enlarge Table 1.
One possible complication with bulk density measurements is the effect of the porosity of the particles themselves; so, to avoid ambiguity, it is worthwhile stating whether the bulk density value is inclusive or exclusive of closed pores. Confusion could arise if the method of determining particle density does not take account of internal voids. (Using a helium pycnometer, which determines particle density by a measure of displaced gas, may be advisable when porosity is a factor. The gas generally can penetrate open pores as long as these are not comparable in size to the gas molecule but obviously cannot penetrate closed pores.) These differences become important if, for example, we are concerned with surface area available for reaction or the total solids fraction available for reaction.
Flowability based on bulk density
Bulk density measurements have been used to give some qualitative prediction of the flowability or handlability of a bulk solid that is, some estimate of the likely ease or difficulty in dealing with these materials. One such predictor is the often-quoted Hausner ratio:These relatively quick and easy measurements can be effective in giving some indication as to how powders will likely behave but are by no means comprehensive; exercise some caution if relying only on this information.
Angle of repose
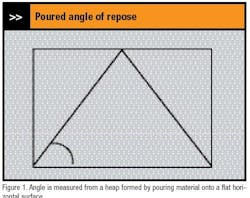
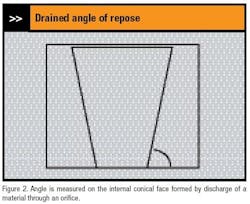
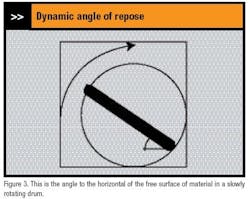
Another parameter that is used to determine flowability is the angle of repose, which is defined as the angle of the free surface of a heap of particulate material to the horizontal plane. Unfortunately, we are faced with the same problem that we were with bulk density the angle of repose is not a constant for a given material and depends upon the method of heap formation. There again are two measurements commonly quoted: the poured angle of repose and the drained angle of repose. The poured angle of repose is the angle measured from a heap formed by pouring material on to a flat horizontal surface (Figure 1). The drained angle of repose is the angle measured on the internal conical face that has been formed when material is drained from a orifice on the flat horizontal bottom of a container (Figure 2). A third angle of repose that you may come across is the dynamic angle of repose, which is the angle to the horizontal of the free surface formed in a relatively slowly rotating drum (Figure 3).
Be aware of several things when using angles of repose: First, the angle formed will depend upon the details of the formation process. For example, the fall height for the poured angle or the orifice size for the drained angle will influence the angle. Therefore, the angle measured is not independent of the measuring apparatus. Second, the same material tested using the three techniques will give a different angle for each (Table 2). The measurements only can be reliably made when using powders that are free-flowing to slightly cohesive and are fairly homogenous. Materials that are a mixture of components or that have a wide size distribution will give angles that are difficult to determine and suffer low repeatability. There also are some uncertainties based on the fundamental physics of the problem, relating to stress history and avalanche behavior [2, 3].
Click to enlarge Table 2.
Despite these difficulties, the angle of repose in whatever form can be a useful tool to rank materials. As a rough guide, the relationship between the angle of repose and flowability often follows the structure below:
Angle of repose, degrees Flowability
25-30 very free-flowing
30-38 free-flowing
38-45 fair flowing
45-55 cohesive
>55 very cohesive
This classification allows us to make some judgement on the likely flow behavior of a material but has very limited value for equipment selection and design. In particular, it is a mistake to use the angle of repose in an estimate of the wall angle required for the converging section of a hopper. However, the angle of repose can serve in some cases to estimate the surcharge (the material at the top of a hopper which forms a heap) in a storage vessel or the ground area requirements when forming a stockpile.
Rough guidance
The Compressibility Index and angle of repose both give some indication of flowability under different flow conditions, although the applied stresses in both cases can be considered to be relatively low. There is no obvious benefit in combining both test results into a single index value; both may be usefully applied separately to benchmark or rank materials based on known plant performance. For example, if you have experience that a material with a particular flowability value passes through a chute or indeed an entire process without difficultly, then you may expect that a different material with the same flowability value also will not cause problems. (Most times, you will be correct.) However, a material with a worse flowability value needs be treated with more caution. Plants suffering from poor performance require more detailed testing to establish the cause(s) of the flow difficulties or product hangups.
The material in this article has been extracted and adapted from the authors recent book Characterisation of bulk solids [1].
Dr. Don McGlinchey is a consulting engineer at Glasgow Caledonian Universitys Center for Industrial Bulk Solids Handling, Glasgow, Scotland. E-mail him at [email protected].
References
1. McGlinchey, D., Characterisation of bulk solids, Blackwell Publishing, Oxford, U.K. (2005).
2. Duran, J., Sands, powders and grains an introduction to the physics of granular materials, Springer-Verlag, New York (2000).
3. Nedderman, R.M. Statics and Kinematics of Granular Materials, Cambridge University Press, Cambridge, U.K. (1992).