Correctly Evaluate Project Economics

For a producer, CCOP is an important measure to assist in evaluating new investments, producing new products from existing equipment, setting operating budgets or justifying process changes. The biggest drawback of CCOP is that it doesn’t include capital costs; it only is useful to help gauge the effects of process enhancements, operational changes, improvements in efficiency or other process alterations that do not require substantial investment. NPV allows ranking options according to net profits, which is reasonable when comparing projects of similar size and scope. Any project’s NPV always can be increased by expanding the project’s size or scope; hence, using NPV to compare large and small projects requires caution.IRR gives a ranking of investments according to their financial yield. IRR enables comparing the performance of capital for different projects, independent of the amount of investment, the life of the plant or the actual interest rates prevailing at any time. IRR is more useful than NPV when comparing projects of different size.

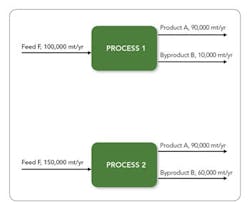
Figure 1. For the same output of Product A, Process 2 requires far more feed.

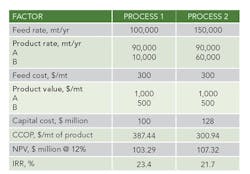
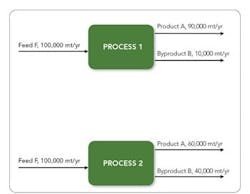
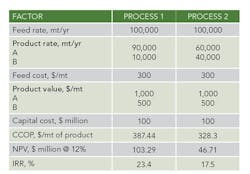
When evaluating different process technologies for a project, the designers select the option that has lowest CCOP, highest NPV or greatest IRR. These methods can be very sensitive to certain starting assumptions, potentially causing a less economical option to be selected. The following example illustrates this.A TELLING EXAMPLEConsider two processes to make the same products, A and B, from Feed F. Process 1 has 90% selectivity to A while Process 2 has 60% selectivity to A. Figure 1 shows the feed and product rates for making 90,000 mt/yr of A.Let’s suppose the capital cost (capex) of Process 1 is $100 million. We then can estimate the capex of Process 2 to be $128 million by applying the 6/10 rule, which assumes the processes have the same capital cost scaled to feed flow rate.C = C₀ (Feedrate_{Process 2} /Feedrate_{Process 1})^0.6where C₀ is the capex of Process 1 and C is the capex of Process 2.A summary of feed and product flow rates and their respective prices for Process 1 and Process 2 appears in Table 2.

Calculating CCOP, NPV and IRR using the definitions from Table 1 and the prices from Table 2 gives the results summarized in Table 2. Process 2 has lower CCOP and higher NPV than Process 1 but Process 1 has greater IRR than Process 2. Thus, if we used CCOP or NPV as the criterion, we would select Process 2 as superior.

Figure 2. For the same feed rate, Process 1 produces much less byproduct.

Now, consider the same two processes operated at a constant feed rate of 100,000 mt/yr (Figure 2). Because the feed rates are the same, we can assume the capital costs are as well. Table 3 provides a summary of feed and product flow rates and their respective prices for Process 1 and Process 2.Table 3 shows the calculated CCOP, NPV and IRR for the constant-feed-rate scenario. Process 1 clearly has higher NPV and IRR than Process 2 but Process 2 still has lower CCOP than Process 1. In this case, we would select Process 1 if either NPV or IRR were the economic criterion. The IRR results for both feed rates indicate that Process 1 always is a more economical option than Process 2. However, the CCOP results suggest that Process 2 always is preferable to Process 1. The NPV favors Process 1 at a constant feed rate but Process 2 at a constant product rate. These differences underscore that choice depends upon the basis selected for the evaluation process.

WHICH METHOD TO USE?So, let’s explore what causes these different outcomes and outline the preferred way to choose the basis for economic evaluation during technology selection.CCOP analysis. In the above example, Process 2, which makes a greater amount of byproduct than Process 1, has lower CCOP because the price of the byproduct ($500/mt) is much higher than that of the feed ($300/mt). In fact, Process 2 will have a better CCOP under any situation in which the byproduct value can offset the higher capital cost of Process 2. As shown in Figure 3, this would be true for any byproduct value greater than $350/mt. The way CCOP is calculated strongly favors processes that form byproducts (i.e., non-selective processes) as long as the byproduct value exceeds the feed value. A byproduct that has less value than the main product reduces the IRR of the project and also may decrease the NPV if the amount of feed available is restricted. So, when using CCOP as the economic tool, byproduct price markedly influences the decision and the designers might end up choosing a less economical option. From this analysis, we can conclude that CCOP is not a good economic tool when evaluating process technologies that make substantial amount of byproducts whose price is higher than the feed but lower than the main product.

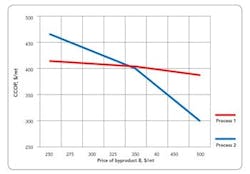
Figure 3. A byproduct price of $350 or more favors Process 2.

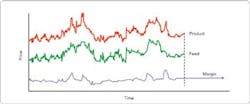
NPV analysis. As noted earlier, NPV increases with project size and, so, designers must exercise caution when using NPV as the economic criterion to compare large and small projects. Consider the examples summarized in Tables 2 and 3. At a constant product rate, Process 2 has the higher NPV because its poor selectivity leads to a larger plant and project size. However, at a constant feed rate, Process 1, which has better selectivity for the main product, has higher NPV. Such a discrepancy always will occur if the economic value of byproduct relative to feed covers the incremental capital cost due to the increase in plant size. The project becomes larger, requiring more capital and generating a less efficient return on investment, but the NPV increases. To avoid this particularly dangerous trap, never rely solely on NPV in evaluating processes that have different selectivity for the main product.IRR analysis. Because IRR is not as sensitive to the scale of the project and allows ranking investments based on their financial yield, it gives the same order regardless of whether the evaluation is conducted at a constant-product or a constant-feed rate. Moreover, IRR is not biased by process inefficiencies that result in a larger plant size or production of byproducts with lower value than the main product. In the above example, Process 1 has higher IRR than Process 2 under all circumstances.Using IRR also can help eliminate the challenge of determining the appropriate discount rate, or interest rate, for the investment. Only when the IRR lands near the hurdle rate for the project is more scrutiny typically required.From this analysis, we clearly can say that IRR is a more useful method than NPV or CCOP for selecting a process flowscheme or project. Also, it provides the true economic advantage (∆IRR between Process 1 and Process 2) when the economic evaluation is conducted at a constant feed rate. In many cases, upstream process conditions restrict the feed supply; however, even for an unconstrained feed supply, a constant feed basis still is preferable because it puts all process schemes (or projects) on the same basis and eliminates any biases due to project size or production of less valuable byproducts.

Figure 4. Margin between product and feed prices varies far less than the prices themselves.

CONSISTENT PRICE FORECASTAn accurate project evaluation depends upon the choice of appropriate prices for the product, feed and utilities. In most cases, after the initial design and construction phase, a plant typically will operate for more than twenty years — so, the prices used in the economic analysis should reflect those forecast over the life of the project. As described in “Chemical Engineering Design” [1], most price forecasts are based on historic price data and several methods can provide predictions of commodity prices. These methods include linear regression of past prices, nonlinear models of price behavior and forecasting of margins. Linear regression of past prices is a good method for capturing long-term trends but can give misleading results depending upon the start date chosen. Nonlinear models can handle commodity prices that exhibit cyclic behavior. Unfortunately, both the amplitude and frequency of the price peaks usually vary somewhat erratically, making it difficult to fit the cyclic price behavior with simple wave models or even advanced Fourier transform methods. Another approach is to recognize that the feed and product prices usually are linked closely, because increases in feed costs usually result in product price rises. Therefore, although feed and product prices both may vary, the gross margin exhibits much less variation and can be forecast more reliably (Figure 4) [1]. The fuels and petrochemicals industry heavily rely on margin forecasts because it is much easier to predict the variation in margins than the underlying variation in the price of crude oil and natural gas. For project evaluation, showing that the prices used for economic analysis are realistic and consistent with consensus views of the market usually suffices. The process industries rely on a variety of utilities, such as steam, process water, cooling water, electricity and nitrogen. Plants usually generate these utilities using the most economical fuel available at the site; typical fuels include fuel gas, natural gas, fuel oil and liquefied petroleum gas. The marginal costs of utilities are based on the marginal cost of fuel. Using fuel equivalents (FEs) enables calculating the marginal cost of the fuel on a consistent BTU basis, taking into account both thermodynamic and mechanical efficiencies, and, thus, puts the marginal cost of any utility on a common basis. FE is defined as the amount of fuel energy (typically the lower heating value expressed in BTUs or equivalent) required for producing an amount of utility:FE = (Heat content of fuel consumed)/(Amount of utility produced)The selection of utilities depends upon the heat requirements, the temperature at which the heat is needed, and the availability and price of different fuels or energy sources. It is crucial always to compare the price of each utility on an FE basis. Substantial differences in prices can create an artificial bias in technology selection. For example, a very low price for natural gas and a high price for steam does not necessarily signify that a process using fired heaters instead of steam heaters is preferred. Rather, it could indicate that the site should add boiler capacity.

AROMATICS APPLICATIONLet’s consider the selection of technology for a new aromatics complex to manufacture para-xylene (p-x), an essential precursor to terephthalic acid, which is used to make polyester. It is commercially produced by catalytic reforming, toluene disproportionation and transalkylation and isomerization of other C8 aromatics.We must choose between two aromatic complexes — the first has a p-x selectivity of x% while the second has a selectivity of (x + 5.2)%. Table 4 summarizes feed, product and byproduct rates used in the evaluation for constant-feed-rate and constant-product-rate cases; Table 5 details the prices used. Table 4 clearly indicates that Complex 2, which has greater selectivity to p-x, has higher NPV and IRR than Complex 1. However, the CCOP results suggest that Complex 1 is a better option than Complex 2. This is because Complex 1 produces a lot more byproducts that are valued at a higher price than the feed, albeit at a lower price than p-x. In this case, we would choose Complex 2 if either NPV or IRR were the economic criterion but we might select the less efficient Complex 1 if CCOP were used. Also, the true economic advantage (∆NPV or ∆IRR) of Complex 2 over Complex 1 only shows when the complexes are evaluated at a constant feed rate. Note that the difference in NPV between the two cases is small when they are evaluated on a constant product basis. This is because the capital cost savings of Complex 2 are offset by a smaller project size due to less formation of byproducts. In this case, the more efficient complex still has a higher NPV but the difference is marginal and easily might be missed during evaluation. On a constant-feed-rate basis, the difference between the complexes is more clearly apparent. Note that this example has been simplified for illustrative purposes and that differences between processes in terms of selectivity often are obscured by disparities in utilities and components of fixed costs.

This case study clearly highlights that evaluating the process technologies at a constant feed rate is preferred and that IRR is a better economic criterion than NPV or CCOP.MAKE THE RIGHT CHOICEThe economic analysis of investment decisions in the process industries can be very sensitive to the assumptions made and methods used. Poor assumptions can mask large differences in process performance. As we have shown, it is important to base technology evaluations on: • constant feed rate not constant product rate;• IRR rather than NPV and CCOP;• consistent marginal utility prices; and • consistent price forecasts.

RAJESWAR GATTUPALLI is lead R&D scientist, CLAYTON SADLER is process design development manager, LAURA LEONARD is principal development specialist and GAVIN TOWLER is chief technology officer and vice president at UOP, a Honeywell company, Des Plaines, Ill. Email them at [email protected], [email protected], [email protected] and [email protected].

LITERATURE CITED
1.    Towler, G. and Sinnott, R.,“Chemical Engineering Design,” 2nd ed., Butterworth-Heinemann, Burlington, Mass. (2012).