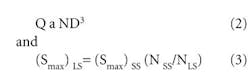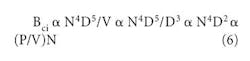Part 1 of this article focused on basic principles and criteria for process design and equipment selection. This final installment will examine the criteria for scaleup, examine use of simulation and highlight some recent innovations in crystallization technology.
An ideal scaleup for any crystallization process would lead to identical levels of supersaturation in all equivalent regions of the laboratory, pilot and plant units. Slurry densities should be the same. Contact times between the crystals and supersaturated liquor should be equal, the Reynolds numbers and Froude numbers of the fluid should be identical, and the contact frequency and energy between crystals, and between the agitator and crystals, should be the same. Ideally, shear forces imparted by the agitator to the slurry and mixing times would be equivalent.
Unfortunately, it's not a perfect world. These goals are often conflicting, even with geometric similarity, and achievement of the same degree of supersaturation and mixing parameters upon scaleup is impossible. In fact, geometric similarity assures that equivalency will never be attained.
Due to conflicting similarity goals, strict geometric scaleup of mixers is restrictive. For example, Reynolds number varies with ND2, the Froude number varies with N2D and the Weber number varies with N2D3. If one of these is used for scaleup, the other two will not scale proportionately. It is better to design a system with more degrees of freedom.
It's important to keep in mind that laboratory and pilot-scale vessels have shorter liquid flowpaths, in both the axial and radial directions, than commercial facilities, yielding reduced turnover times -- typically 1-3 sec. versus 20-60 sec. or more for common commercial equipment. Turnover time is defined as the active slurry volume divided by the axial flow rate generated by the impeller.
An effective way around this problem is to use different geometries for the smaller units. Because the laboratory or pilot facility is so "well mixed," one might consider using a narrower blade and smaller agitator-to-tank-diameter, or D/T ratio, in the small equipment. Narrowing the D/T limits the increase in shear rate variation that occurs if D/T is held constant during scaleup. The reduction in D/T for the pilot plant increases the distribution of shear rates in that vessel and makes the distribution closer to that of the production unit.
If a mixer is scaled up with geometric similarity and constant P/V, the maximum shear rate at the blade increases, being proportional to D1/3, while the average shear rate decreases, being proportional to D-2/3. This maximum shear rate may present a breakage and secondary nucleation problem for some crystallizations.
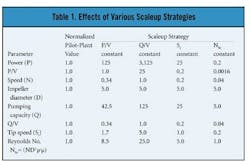
Table 1 presents the results of different agitator scaleup strategies when utilizing geometrically similar vessels. The first column describes a number of pertinent agitation parameters, while the second column normalizes the same data by assigning a value of 1.0 to represent the value that would be found for each in a typical 25-gal. pilot plant crystallizer.
Each of the four remaining columns shows the implications of a chosen strategy for geometric scaleup to a 3,125 gal. full-scale vessel. In carrying out a given strategy, one of the parameters is held constant (e.g., P/V in the case depicted by Column 3). The other values in the column show the effect of that strategy on the other parameters.
Column 3 demonstrates that, if P/V is to be held constant for a geometric scaleup, the agitator speed will drop and the agitator-generated slurry turnover (Q/V) within the vessel will fall off. This criterion yields the same local energy dissipation and, thus, specific power input for micromixing.
With larger vessels, the maximum macroscale shear rate in the impeller zone increases while the average decreases. The maximum increases due to the tip speed, while the average decreases in response to speed. Experiments have shown that scaling on the basis of constant P/V usually results in a decrease in secondary nucleation.
Column 4 assumes holding active volume turnover rate constant, which makes P/V proportional to the square of the impeller diameter, while maintaining constant speed. In this case, the residence-time distribution (RTD) is constant. This causes a large rise in pumping power and yields similar macromixing, constant circulation time and constant pumping rate per unit volume. This choice is impractical, suggesting that a pilot plant's attractive low blend time and circulating time cannot realistically be maintained during geometric scaleup. Severe breakage and secondary nucleation can result.
As indicated in Column 5, when specifying constant tip speed, ST, (therefore constant torque-per-unit-volume for turbulent flow conditions with Newtonian fluids) the maximum impeller zone shear rate remains constant, but the turnovers drop significantly, along with the P/V. It yields equal bulk fluid velocities and can be considered as an approximate scaleup for constant mesomixing, since blending of incoming reactant with bulk or a second reactant is closely linked to shear in the mixing zone. Some experimental evidence indicates that scaleup on the basis of tip speed will result in a decrease in secondary nucleation rate.
Column 6 shows that it is totally impractical or impossible to keep the impeller Reynolds number constant, because the P/V value and the turnover would become unrealistically low.
In conclusion, Table 1 shows that for any realistic scaleup:
The above analysis predicts that secondary nucleation will be reduced when scaling up on the basis of either constant P/V or constant ST, with the reduction being greater for the latter. However, turnovers and blending are further reduced when employing the tip speed criteria. If constant tip speed is selected, one must make sure that the velocities are adequate to maintain solids suspension and, especially for precipitations, that the micromixing is sufficient to reduce inhomogeneities that result in high levels of supersaturation. If not, primary nucleation becomes possible.
Minimizing nucleation
In small vessels of around 50-100 gal., most secondary nuclei are due to crystal contact with the agitator and walls. As the vessel size increases, there are relatively more crystal-to-crystal collisions, due to the reduced surface area per unit volume.
Vessels with high slurry density (usually 25 percent solids or more) have nucleation due to crystal-to-crystal contact. For low slurry densities (usually
2%-5%), crystal-to-impeller contacts are the most important source of nuclei. It has been found that P/V is extremely important for both of these secondary sources of nucleation. Proper agitator design is required.
Primary nucleation
where Smax is the supersaturation and Qc is the rate of circulation.
The required minimum circulation for the small-scale vessel can be estimated by varying the speed and observing the point of primary nucleation. For geometrically similar systems:
which qualitatively indicates equal speeds on scaleup if the small-scale unit is at the critical point. This results in high power requirements to avoid primary nucleation, which negatively impacts breakage and secondary nucleation. Thus, there is a likely choice between the possibility of primary nucleation and low secondary nucleation rates or having high levels of secondary nucleation and breakage while minimizing primary nucleation.
Secondary nucleation
Crystal-impeller
where Bci is the nucleation rate and MT is the slurry density.
For a given crystallizer where Smax is held constant, Q must be constant Therefore, ND3 is constant or N a D-3. Thus,
The use of a large, slow speed impeller with a high NQ relative to its NP, such as a hydrofoil, can greatly reduce secondary nucleation.
Geometric similarity
From Equation 6, the following relationships result for the chosen scaleup strategy.
Crystal-crystal impacts become controlling at high slurry densities and large scales. In such cases, it can be qualitatively shown that:
where BCC is the nucleation rate and dp is the particle size.
Again, this relationship emphasizes the need to minimize the P/V and, in particular, the N since:
Low shear, efficient hydrofoils will assist in this effort. Moderate increases in N can result in large increases in secondary nucleation. One can sometimes increase the particle size by increasing the slurry density above its natural make. This is due to the decrease in supersaturation resulting from the higher crystal surface area. However, the increased solids loading may produce more secondary nucleation.
Attrition and breakage
The most important stresses are impact-induced stresses (e.g., crystal-crystal, crystal-wall and crystal-impeller) and fluid-induced stresses (e.g., shear stress, drag stress and pressure/normal stress). The results of these phenomena are often seen as shards and broken pieces in the crystal slurry.
Simulation and modeling
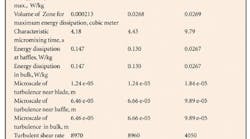
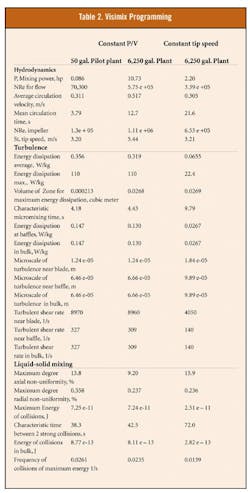
Two cases were analyzed, the first being scaleup at constant P/V and the second being scaleup at constant tip speed, St.
When scaling up with a constant tip speed, as expected, the turbulence parameters predict a significant reduction in energy dissipation rates, turbulent shear rates and an increase in the microscale of turbulence. The turbulence values for scaleup at a constant P/V are quite close to each other.
The hydrodynamic values show the dramatic change in Reynolds number and mean circulation times.
The liquid-solid mixing characteristics predict reduced values for the energy of collision, frequency of collisions and characteristic time between two strong collisions when scaling up on a tip-speed basis. This would predict the expected decrease in secondary nucleation.
A summary of definitions for the predicted parameters is as follows:
1. Maximum value of energy dissipation -- microscale phenomena -- energy dissipation behind the agitator blades -- controls breakage, nucleation and micromixing in this zone.
2. Local values of energy dissipation -- microscale phenomena -- average energy dissipation plus energy dissipation in bulk slurry and at baffle -- controls breakage, nucleation and micromixing in these zones.
3. Characteristic time of micromixing -- time of microscale degradation of non-homogeneous concentrations -- important for precipitations.
4. Shear rates -- characteristic shear rate at the microscale level --scaleup governs mass transport process for growing and dissolving solids.
5. Maximum energy of collisions at zone of maximum turbulence near impeller blades -- collisional energy of particles -- higher values increase breakage and secondary nucleation.
6. Energy of collisions in bulk -- collisional energy of particles -- although less than the maximum value, the large number of collisions in the bulk can affect breakage and secondary nucleation.
7. Frequency of collisions of maximum energy -- predicts how often the slurry particles see the maximum collisional energy -- if higher, more breakage and secondary nucleation.
8. Time between two strong collisions -- average period of uninterrupted crystal growth.
New developments
Sonocrystallization
Ultrasound can also be used to generate secondary nuclei via the impact of the large forces originating from the collapse of cavitation bubbles on or near the crystal surfaces. The technology can influence growth by enhancing mass transfer near the crystal surface. Crystal purity may improve, as crystal surface impurities are preferentially dissolved in the temporary local undersaturated solution, resulting from highly localized heating from cavitation near the crystal surfaces.
It may be possible to dictate the polymorph that is crystallized via this technology, by controlling the supersaturation at the point of nucleation. Commercial units typically operate at around 20 kHz with multiple transducers, each with relatively low power output (approximately 0.1-1.0 W/cm2 at the point of delivery) coupled to the wall of the crystallizer. Average power densities for the mutliple transducers are in the 75-80 W/L range.
CFD
Measurement techniques
Wayne Genck, principal of Genck International, is an industrial consultant in the field of crystallization and precipitation. He can be reached at (708)748-7200.